A1127 ZigZagging on a Tree (30 point(s))

中序+后序=层序

1. 原文

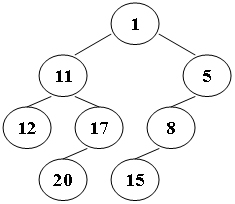
Suppose that all the keys in a binary tree are distinct positive integers. A unique binary tree can be determined by a given pair of postorder and inorder traversal sequences. And it is a simple standard routine to print the numbers in level-order. However, if you think the problem is too simple, then you are too naive. This time you are supposed to print the numbers in “zigzagging order” – that is, starting from the root, print the numbers level-by-level, alternating between left to right and right to left. For example, for the following tree you must output: 1 11 5 8 17 12 20 15.

Input Specification:

Each input file contains one test case. For each case, the first line gives a positive integer N (≤30), the total number of nodes in the binary tree. The second line gives the inorder sequence and the third line gives the postorder sequence. All the numbers in a line are separated by a space.

output Specification:

For each test case, print the zigzagging sequence of the tree in a line. All the numbers in a line must be separated by exactly one space, and there must be no extra space at the end of the line.

Sample Input:

8
12 11 20 17 1 15 8 5
12 20 17 11 15 8 5 1

Sample output:

1 11 5 8 17 12 20 15

2. 解析

中序 左 根 右

后序 左 右 根

getLevel(int inl,int inr,int postr,int deep) 

level[deep].push_back(post[postr])

每层递归添加

最终层按奇偶数输出

 printf("%d", level[1][0]);
for (int i = 2; i <= maxdeep; ++i)
{
	if (i%2==0){} 
   else{}
 }

3. AC代码

#include<cstdio>
#include<vector>
using namespace std;
int in[40]={};
int post[40]={};
int maxdeep=-1;
vector<int> level[40];
void getLevel(int inl,int inr,int postr,int deep){
	if (deep>maxdeep)
	{
		maxdeep=deep;
	}
	if (inl>inr)
	{
		return;
	}
	level[deep].push_back(post[postr]);
	int i=0;
	for (i = inl; i <= inr; ++i)
	{
		if (in[i]==post[postr])
		{
			break;
		}
	}
	getLevel(inl,i-1,postr-(inr-i)-1,deep+1);
	getLevel(i+1,inr,postr-1,deep+1);

}
int main()
{
	int n;
	scanf("%d",&n);
	for (int i = 0; i < n; ++i)
	{
		scanf("%d",&in[i]);
	}
	for (int i = 0; i < n; ++i)
	{
		scanf("%d",&post[i]);
	}
	getLevel(0,n-1,n-1,1);

	printf("%d", level[1][0]);
	for (int i = 2; i <= maxdeep; ++i)
	{
		if (i%2==0)
		{
			for (int j = 0; j < level[i].size(); ++j)
			{
				printf(" %d",level[i][j]);
			}
		}else{
			for (int j = level[i].size()-1; j >=0 ; j--)
			{
				printf(" %d",level[i][j]);
			}
		}
	}
	return 0;
}